Pressure Measurement
Application Note: Basics of Air Velocity, Pressure and Flow
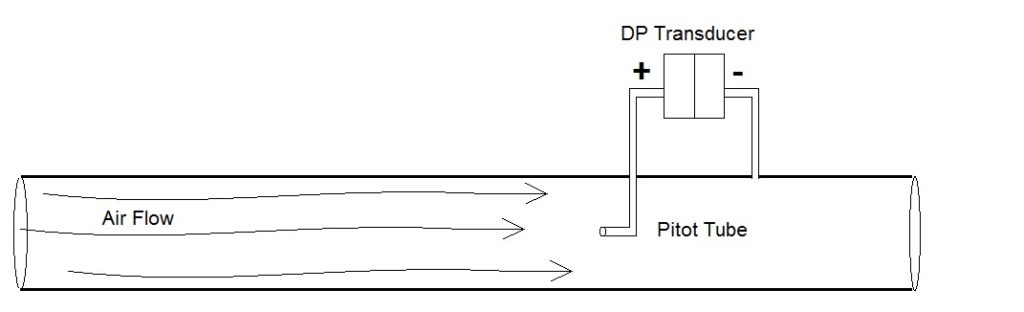
Air velocity can be measured by sensing the pressure produced by the movement of the air. This application note will describe the basic relationships between air velocity and the pressure…
Read More